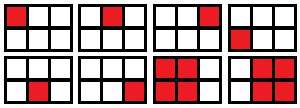
2599.Spongebob and Squares
Time Limit: 1s Memory Limit: 256MBSpongebob is already tired trying to reason his weird actions and calculations, so he simply asked you to find all pairs of n and m, such that there are exactly x distinct squares in the table consisting of n rows and m columns. For example, in a 3 \times 5 table there are 15 squares with side one, 8 squares with side two and 3 squares with side three. The total number of distinct squares in a 3 \times 5 table is 15+8+3=26.
Input Format(From the terminal/stdin)
Input The first line of the input contains a single integer x (1 \le x \le 1018)- the number of squares inside the tables Spongebob is interested in.
Output Format(To the terminal/stdout)
Output First print a single integer k- the number of tables with exactly x distinct squares inside.Then print k pairs of integers describing the tables. Print the pairs in the order of increasing n, and in case of equality- in the order of increasing m.
Sample Input 1
26\n
Sample Output 1
6 1 26 2 9 3 5 5 3 9 2 26 1\n · \n · \n · \n · \n · \n · \n
Hints
In a 1 \times 2 table there are 2 1 \times 1 squares. So, 2 distinct squares in total.  In a 2 \times 3 table there are 6 1 \times 1 squares and 2 2 \times 2 squares. That is equal to 8 squares in total.
In a 2 \times 3 table there are 6 1 \times 1 squares and 2 2 \times 2 squares. That is equal to 8 squares in total.